Harmonising The Major Scale
Harmonising the major scale
As we all know, the major scale plays a central role in Western music. Almost all of the music we listen to relates to the major scale in one way or another and it is the mother of all of the most common chords and modes that we deal with as musicians on a day to day basis.
Really getting to know the scale and its relation to the chords we use is an essential basis to dive deeper into the world of Jazz harmony. Harmonising the major scale using triads and seventh chords is a fantastic way of opening up new insights into its structure and harmonic possibilities.
Harmonising the major scale using triads
Triads are made up of three notes – the root (1), the third (3) and the fifth (5).
The quickest way of finding the right notes for your triad is going up its respective scale in thirds, which is most easily done by skipping every other note of the scale, and stacking those notes on top of the root.
Within the C major scale for example, the third of C would be E (we skipped the D) and by adding yet another third (skipping the F) we’d get to G, completing our C major triad:
The Harmonised Major Scale
CDEFGABC
If we’d now want to construct a triad using the same scale but starting on D, we’d simply do the same thing going up from there, which would get us the notes D – F – A (a D minor triad).
CDEFGABC
This way we can go through all of the notes of the major scale and assign a chord to each one of them. If you hear someone talk about a “I – IV – V (read: one, four, five) progression”, they are simply referring to the chords that you will find on the first, fourth and fifth note of the scale. In our example of C major, this would be a C, F and G Chord.
Chord qualities
Within chords based on the major scale, thirds can be either major or minor (3 / b3) and fifth can be either perfect or diminished (5 / b5). The type of third/fifth we end up with is determined by the interval (the amount of semitones) between a note and the root of the chord. For a full list of intervals, please have a look at the interval chart at the bottom of this article.
The combination of intervals within a chord determines the type, or quality of the chord. When harmonising the major scale using triads, we will encounter three types of chords:
Major: 1–3–5 Minor: 1 – b3 – 5 Diminished: 1 – b3 – b5
If you keep going through the scale as we did above, you will end up with the following chords for each step (degree) of the scale:
|
Degree |
I |
II |
III |
IV |
V |
VI |
VII |
|
Chord type |
Major |
Minor |
Minor |
Major |
Major |
Minor |
Diminished |
|
In C major |
C |
Dm |
Em |
F |
G |
Am |
Bdim |
Here’s a way of playing through the harmonised C major scale using triads:
Seventh Chords
So called seventh chords consist not of three, but of four notes. The first three are identical to the notes within a triad (1 – 3 – 5). In order to construct a seventh chord, we simply stack yet another third on the top of the triad, giving us a chord that consists of a root, a third, a fifth and a seventh.
Just like the third, the seventh can be either major or minor (maj7 / b7), depending on whether it is ten or eleven semitones away from the root (see interval chart).
When harmonising the major scale using seventh chords, we will encounter four different kinds of chords:
Major 7 (written maj7): Dominant 7 (written dom7 or 7): Minor 7 (written m7):
Half diminished (written m7b5):
Constructing seventh chords
1 – 3 – 5 – maj7 1 – 3 – 5 – b7
1 – b3 – 5 – b7 1 – b3 – b5 – b7
Using the same method as we did with our triads, let’s have a go at finding the seventh chords of each note within our example scale C major:
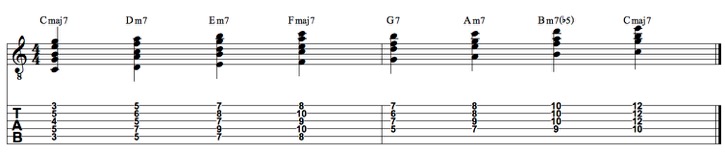
Harmonising the major scale
As we already know, the C major triad consists of the notes C – E – G. If we then add another third to that triad (going up from G, skipping the A) we’ll get the extra note B:
CDEFGABC
The chord we end up with now consists of 1 – 3 – 5 – maj7, thus making it a Cmaj7
chord.
Let’s try the same for D:
CDEFGABC
This time we end up with: 1 – b3 – 5 – b7, a Dm7 chord.
You can go through the whole scale in this fashion and will eventually end up with a seventh chord for each note of the scale:
|
Degree |
I |
II |
III |
IV |
V |
VI |
VII |
|
Chord type |
maj7 |
m7 |
m7 |
maj7 |
dom7 |
m7 |
m7b5 |
|
In C major |
Cmaj7 |
Dm7 |
Em7 |
Fmaj7 |
G7 |
Am7 |
Bm7b5 |
Here’s a way of harmonising the C major scale on your guitar, using seventh chords. Please note that rather than sticking to the 1 – 3 – 5 – 7 order, we are now inverting the chord to a 1 – 5 – 7 – 3 structure, with the first four chords having an optional doubled 5 on top of that. Inverting chords and doubling notes is a common practice on the guitar and other harmony instruments.
You will find the most common chord shapes for maj7, min7 and dom7 chords at the bottom of this article. Try harmonising different major scales using those shapes!
It’s also a lot of fun and great practice to come up with chord progressions
(e.g. I – VI – II – V) and transpose them through different keys.
Seventh Chord shapes with their root note (square) on the E- and A-String
Interval chart
|
Semitones |
Interval |
|
0 |
Perfect Unison |
|
1 |
Minor Second |
|
2 |
Major Second |
|
3 |
Minor Third |
|
4 |
Major Third |
|
5 |
Perfect Fourth |
|
6 |
Diminished Fifth |
|
7 |
Perfect Fifth |
|
8 |
Minor Sixth |
|
9 |
Major Sixth |
|
10 |
Minor Seventh |
|
11 |
Major Seventh |
|
12 |
Perfect Octave |

2 Octave Major scale
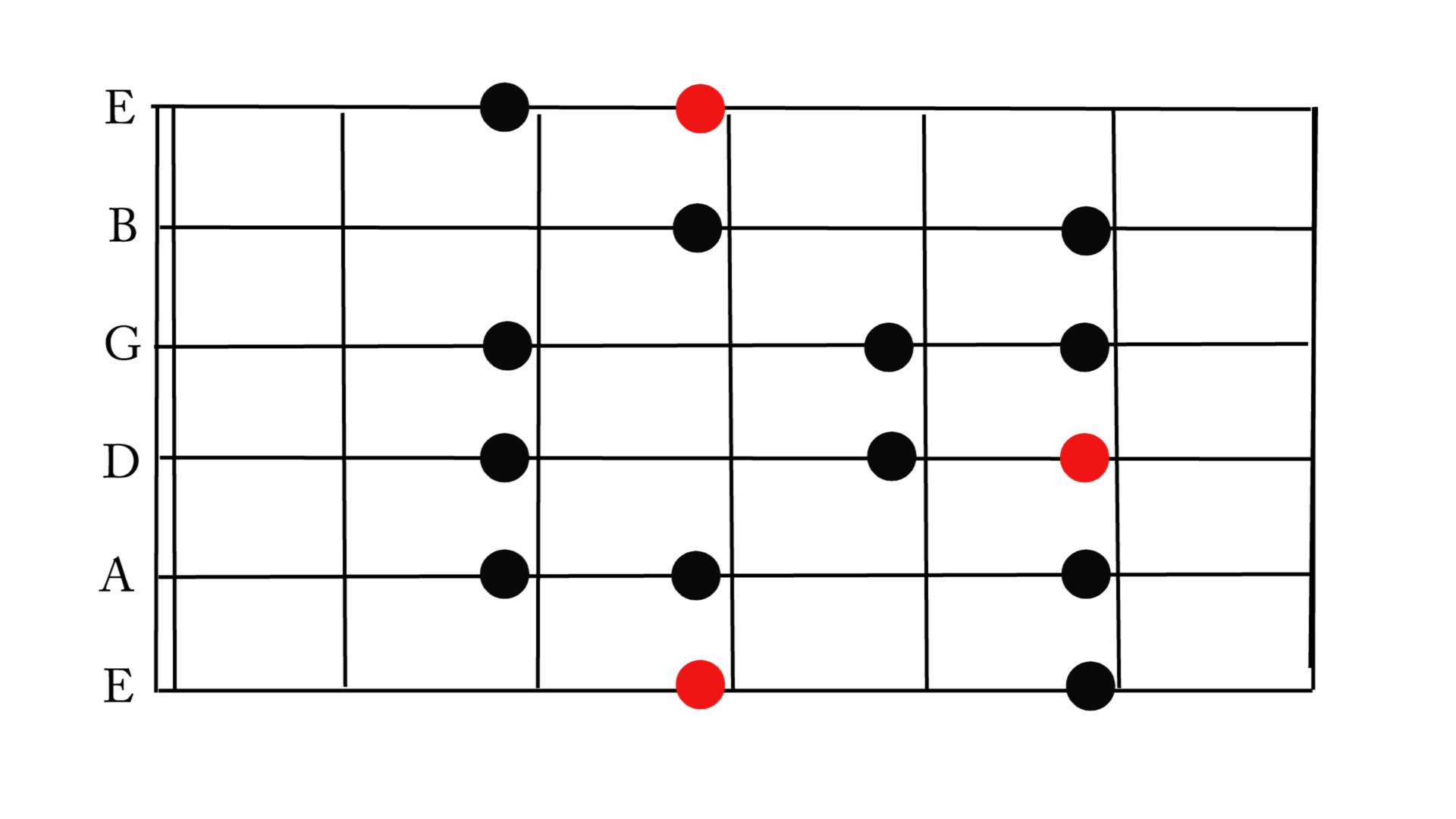
Major Scale
